Calculating Standard Enthalpy of Reaction

Standard Enthalpy of Formation

Standard Enthalpy of Reaction (ΔH_rxn­) is the amount of heat absorbed (+ΔH value) or released (-ΔH value) that results from a chemical reaction.

ΔH_rxn is calculated using the standard enthalpy of formation for each compound or molecule in the reaction. The enthalpies of all reactants are added and the sum of the enthalpies of the reactants are subtracted from that value.

 Σ (ΔH° products) – Σ (ΔH° reactants)

 An important note that the enthalpy values found in most reference tables are for 1 mole of that substance in units of kilojoules (kJ)! That means that is the energy associated with 1 mole of that substance forming; therefore, you need to multiply that value by the number of moles of that substance in the given reaction to find the TOTAL enthalpy from that substance.

A substance may also be listed more than once, in each of its physical states. Recall that enthalpy is a state function, which means it has various values at different physical states. The symbol knot (°) denotes standard conditions of 25°C and 1 atm of pressure.

For reaction:  

aA + bB —-> cC + dD

Equation Would Be:

ΔH°_rxn = [ (c)(ΔH_f°_C) + (d)( ΔH_f°_D) ] –  [ (a)(ΔH_f°_A)+ (b) (ΔH_f°_B) ]

Where:

c = # mols of product C          ΔH_f°_C = enthalpy of  C

d = #mols of product D          ΔH_f°_D = enthalpy of  D

a = #mols of reactant A          ΔH_f°_A = enthalpy of  A

b = #mols of reactant B          ΔH_f°_B = enthalpy of  B

Example:

What is the standard enthalpy of the reaction (ΔH°_rxn) for the combustion of propane using the following balanced chemical equation? Standard enthalpy values for reactants and products are given in the table below.

C3H8 (g)+ 5O2 (g)  —–> 3CO2 (g) + 4H2O (g)

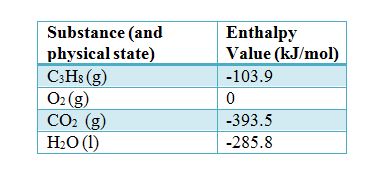
ΔH°_rxn= [ (3) (ΔH°_CO2) + (4) (ΔH° _H2O)] – [ (1) (ΔH°_C3H8) + (5) (ΔH°_O2)

ΔH°_rxn= [ (3 mol) (-393.5 kJ/mol) + (4 mol)  (-285.5 kJ/mol) ] – [ (1 mol) (-103.9 kJ/mol) + (5 mol) (0 kJ/mol)

ΔH°_rxn= [-1180.5 kJ + (-1142 kJ)] – [-103.9 kJ]

ΔH°_rxn= (-2322.5 kJ) + 103.9 kJ

ΔH°_rxn= -2218.6 kJ

Does this ΔH_rxn seems reasonable? YES! Because it is a – ΔH_rxn value which makes this reaction exothermic. Combustion reactions indeed produce and release heat.

Note: Substances which naturally occur or appear in nature have a ΔH value of zero. Examples include naturally occurring elements such as aluminum (Al) and carbon (C) in its pure graphite form, as well as diatomic gases such as oxygen (O­₂) and nitrogen (N₂). Why? Because they already exist in nature so no energy is expended to form them.